If you connect a 12-volt battery to a 4-ohm lamp, 3 amps of current will flow through the circuit by Ohm’s Law, V=IR. Power = VI = 36 watts will be dissipated by the lamp. If you add a 2-ohm resistor in series with the lamp, the resistances add to 6 ohms, the current is 2 amps and the circuit thus dissipates 24 watts. The voltage across the resistor is 4 volts so it dissipates 8 watts and the lamp gets 8 volts and dissipates 16.
What if you had a gadget with a resistance of negative 2 ohms? Let’s replace the resistor with it and see what happens: total resistance 4-2=2 ohms, current 6 amps, voltage across the lamp 24 volts, voltage across the resistor minus 12 volts. The lamp is dissipating 144 watts, of which the battery at 12V*6A is only supplying 72 — the resistor is supplying, rather than dissipating, the other 72.
It’s fairly easy to create a circuit that exhibits negative resistance using op amps:
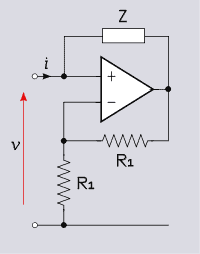
… but why would you want to?
After all, the energy from the op amp isn’t free; it draws its power from somewhere. But suppose you had a circuit element that had some source of energy, say, it was photovoltaic and sat in the sunlight. Then acting like a negative resistance might be a useful way to act in a circuit.
For example, a sheet of resistive material when considered as a 2-D shape has a very simple and regular resistive behavior: any square shape of it has the same resistance, no matter what size. (Resistivity of layers in VLSI is measured in “ohms per square”, for example, a parameter dependent on the material but not the size.) Essentially the wider the path, the lower the resistance, and the longer, the higher, so in any square, they cancel.
Now suppose you had nanofabricated modules, say 10 microns in diameter, dispersed as an emulsion in a fluid, which you then put in an ordinary paint sprayer and applied to the roof of your house. The incalculably complex network of nanomodule touching nanomodule across the house adds up to the nice even continuous sheet approximation and you just count squares from electrode to electrode.
Suppose your house load (all your appliances, in parallel) is 10 ohms and you’d like to match it with a -10 ohm solar sheet. Your roof is 20 feet wide and 40 feet long — 2 squares — so get -5-ohm-per-square paint and put electrodes at each end. If the hardware store only carries -20-ohm-per-square paint, put the electrodes along the sides so the squares are in parallel.
You can’t, however, put the roof in series with your house load as in the lamp example above. Consider what happens if the negative resistance just matches the load: 4 ohm lamp, -4 ohm resistor, total resistance 0, current at 12V infinity. (It’s trying to simulate a superconductor.) Woops!
What you do is what any sane electrician would have done in the first place, which is put the roof in parallel with the house load. Then if the resistances match exactly, the result is infinite rather than 0 resistance, and 0 rather than infinite current (to be drawn from the grid). Which is roughly how solar panels work anyway. (I’m pretending it’s DC, but the AC case is similar if a bit more, um, complex.)
Footnote: This only scratches the surface of solar paint. For example, loops of negative resistance would act to amplify magnetically induced eddy currents, wasting power. It would be an amplifying mirror for electromagnetic radiation. So in practice, you’d have to engineer and tune a whole range of electromagnetic properties at many frequencies.
